The modern electronic calculator allows you to perform a wide range of calculations quickly, easily and securely. That's not the case with its mechanical predecessors. They were usually only good for one or a very limited number of operations.
It is not surprising, therefore, that various calculating aids were combined. A slide adder is very handy for adding and subtracting, but rather clumsy for multiplying. Troncet therefore added a book of tables to his arithmographe. Tables by Jean Bergmann, Wilken Wilkenson, ... were equipped with a small slide adder.
The sorotaku by SHARP can rather be seen as a way of cushioning the sudden change from calculating with the abacus to the electronic calculator.
The combination of two or more of the same or similar machines could also contribute to faster calculation.
ARITHMOGRAPHE TRONCET, Libraire Larousse (Paris, France), 1889 … 1914
This "early" version of the Troncet Arithmographe already includes a book of tables with multiplications from 0 to 999. A slide adder allows you to just add and subtract quickly. Intermediate results could be written on the black areas below or above the result windows and on the front and rear pages of the book of tables.

Collection: E. Smet (item 141)
Universal Calculator, Jean Bergmann - CBR (Berlin, Germany), 1923
The red stamp on the title page shows how important it was to protect products (patents, ...). Sometimes the resulting animosities led to a lawsuit, the result of which was communicated to customers in this way.

Collection: E. Smet (item 129 & 152)
ADDIATOR ADDIMULT Multi-Divi (Vikarby, Sweden and Berlin, Germany), 1946
In addition to multiplication tables, the book contains tables for calculating percentages, squares, square roots and logarithms. The book was printed in Sweden by Wilken Wilkenson for Addiator.

Collection: E. Smet (item 52)
SHARP EL-428 (Japan, South-Korea), 1978-1985

Collection: E. Smet (item 287)
A.W. FABER-CASTELL-Addiator 1/22A (Berlin & Stein, Germany), after 1935
This type of slide rule was specially designed for merchants trading with the USA or Great Britain. A very compact Addiator Universal is built into the back of the slide rule.

Collection: E. Smet (item 364)
FABER-CASTEL 67/54Rb (Wolfach & Stein, West-Germany), 1958-1976
In the second generation of hybrid calculating aids, the plastic slide rule formed the back cover of the metal slide adder.

Collection: E. Smet (item 364)
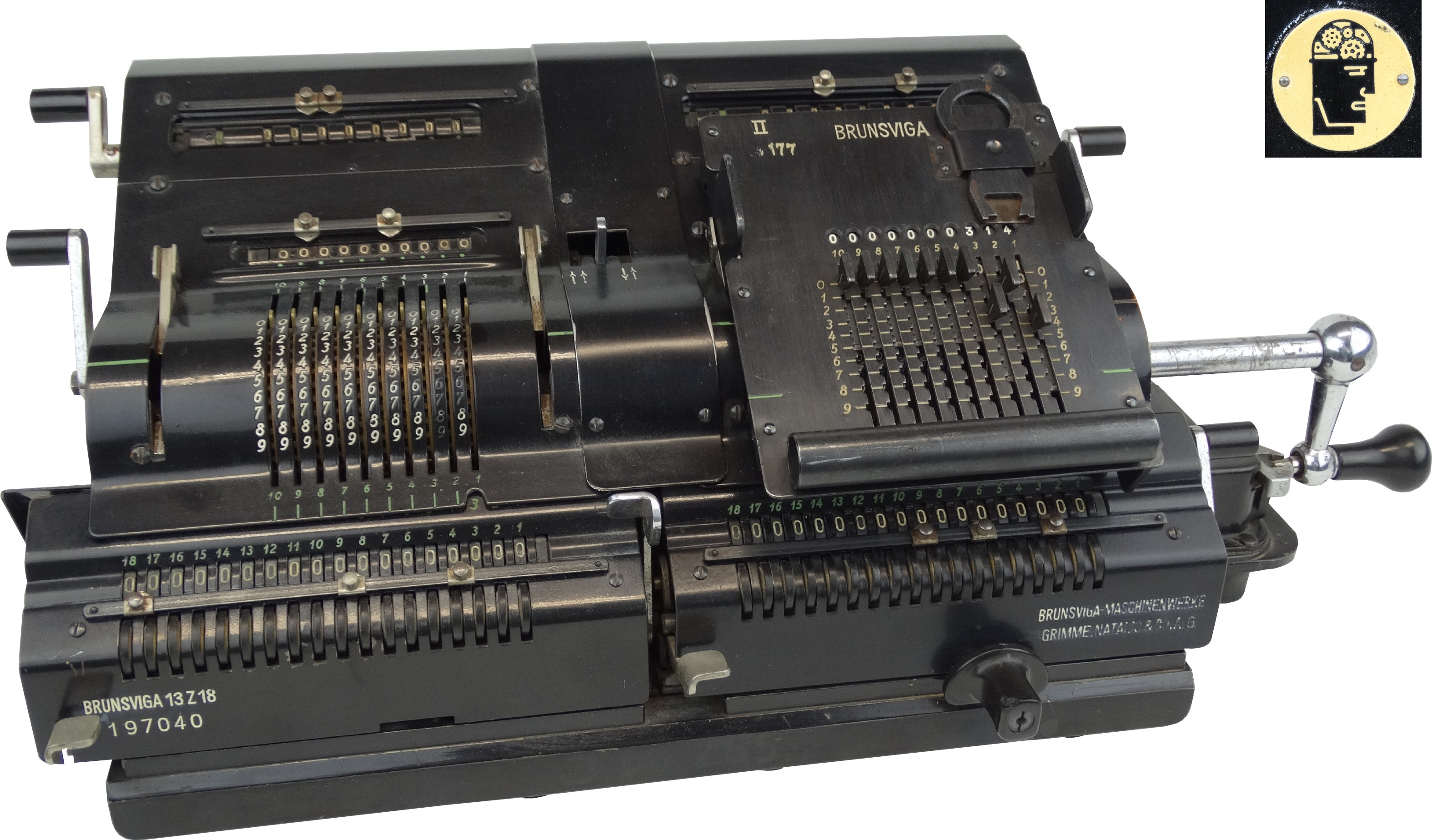
Brunsviga D13Z-1 (Brunsviga-Maschinenwerke Grimme, Natalis & Co A.-G., Germany) ca. 1939
This coordinate calculator was designed to save time when calculating coordinate transformations in a two-dimensional plane, e.g. transforming coordinates for a cadastre, surveying, or calculating trajectories or ranges for artillery. It simply consists of two pinwheel machines on the same main shaft, so that the numbers set in both machines can be multiplied by a common factor. Between the two machines is a drive with two sets of conical gears that allow the direction of rotation of the left machine to be reversed with respect to the right one - important, of course, if your y-coordinate has a minus sign.
Very often these coordinate transformations used the same returning factors. For this reason, the machine is equipped with a "constant plate". Using the key provided, the plate can be set to a particular constant (e.g. a particular sine or cosine value that is often used in subsequent calculations) - by placing the plate on the rails and pulling it down, the constant in the plate is transferred to the machine's setting register. Having a library of pre-set constant plates could speed up the coordinate transformation work considerably.
To avoid making mistakes, this type of calculation was often done using pre-printed forms that contained some sort of algorithm that the operator could simply follow.
For artillery calculations, a (British) "computing centre" consisted of a corporal, four privates and two calculating machines. A cannon was obviously not fired until both teams had calculated the exact same elevation... an early application of the "four eyes" principle - measure twice, cut once - or in this case, fire once!
Collection: C. Vande Velde